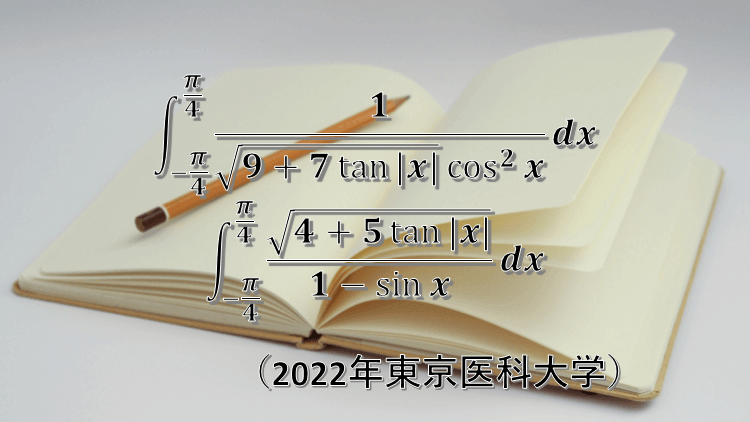
\begin{eqnarray}
&& \int_{-\frac{\pi}{4}}^{\frac{\pi}{4}} \frac{1}{\sqrt{9+7 \tan |x|} \cos^2 x} dx = \fbox{(1)} \\
&& \int_{-\frac{\pi}{4}}^{\frac{\pi}{4}} \frac{\sqrt{4+5\tan |x|}}{1-\sin x} dx = \fbox{(2)}
\end{eqnarray}
【解説】
一見、複雑そうな定積分の計算に見えますが、積分区間が原点を挟んで対称なので、奇関数は省略できます。あとは \( \displaystyle (\tan x)^{\prime} = \frac{1}{\cos^2 x} \) を利用すると、置換積分できます。
【解答】
(1)\( \tan |x|, \ \cos^2 x \) は偶関数だから、積分の中身の関数は偶関数となるので、
\begin{eqnarray}
&& \int_{-\frac{\pi}{4}}^{\frac{\pi}{4}} \frac{1}{\sqrt{9+7 \tan |x|} \cos^2 x} dx \\
&& = 2 \int_0^{\frac{\pi}{4}} \frac{1}{\sqrt{9+7 \tan |x|} \cos^2 x} dx \\
&& = 2 \int_0^{\frac{\pi}{4}} \frac{1}{\sqrt{9+7 \tan x}} \cdot \frac{1}{\cos^2 x} dx \\
&& = 2 \int_0^{\frac{\pi}{4}} (9+7 \tan x)^{-\frac{1}{2}} \cdot (\tan x)^{\prime} dx \\
&& = 2 \left[ \frac{2}{7} (9+7 \tan x)^{\frac{1}{2}} \right]_{0}^{\frac{\pi}{4}} \\
&& = \frac{4}{7} ( \sqrt{9+7 \cdot 1} – \sqrt{9} ) \\
&& = \frac{4}{7} ( 4 – 3 ) \\
&& = \frac{4}{7} \cdots (答)\\
\end{eqnarray}
(2)
\begin{eqnarray}
&& \frac{\sqrt{4+5\tan |x|}}{1-\sin x} \\
&& = \frac{\sqrt{4+5\tan |x|}}{1-\sin x} \cdot \frac{1+\sin x}{1+\sin x} \\
&& = \frac{\sqrt{4+5\tan |x|}}{\cos^2 x} \cdot (1+\sin x) \\
&& = \frac{\sqrt{4+5\tan |x|}}{\cos^2 x} + \frac{\sqrt{4+5\tan |x|}}{\cos^2 x} \cdot \sin x
\end{eqnarray}
ここで、\( \tan |x|, \ \cos^2 x \) は偶関数、\( \sin x \) は奇関数だから、
\( \displaystyle \frac{\sqrt{4+5\tan |x|}}{\cos^2 x} \) は偶関数、\( \displaystyle \frac{\sqrt{4+5\tan |x|}}{\cos^2 x} \cdot \sin x \) は奇関数となる。よって、
\begin{eqnarray}
&& \int_{-\frac{\pi}{4}}^{\frac{\pi}{4}} \frac{\sqrt{4+5\tan |x|}}{1-\sin x} dx \\
&& = \int_{-\frac{\pi}{4}}^{\frac{\pi}{4}} \left( \frac{\sqrt{4+5\tan |x|}}{\cos^2 x} + \frac{\sqrt{4+5\tan |x|}}{\cos^2 x} \cdot \sin x \right) dx \\
&& = 2 \int_{0}^{\frac{\pi}{4}} \frac{\sqrt{4+5\tan |x|}}{\cos^2 x} dx \\
&& = 2 \int_{0}^{\frac{\pi}{4}} \frac{\sqrt{4+5\tan x}}{\cos^2 x} dx \\
&& = 2 \int_{0}^{\frac{\pi}{4}} (4+5\tan x)^{\frac{1}{2}} \cdot (\tan x)^{\prime} dx \\
&& = 2 \left[ \frac{1}{5} \cdot \frac{2}{3} (4+5\tan x)^{\frac{3}{2}} \right] \\
&& = \frac{4}{15} \left( 9^{\frac{3}{2}} – 4^{\frac{3}{2}} \right) \\
&& = \frac{4}{15} (27-8) \\
&& = \frac{76}{15} \cdots (答)
\end{eqnarray}